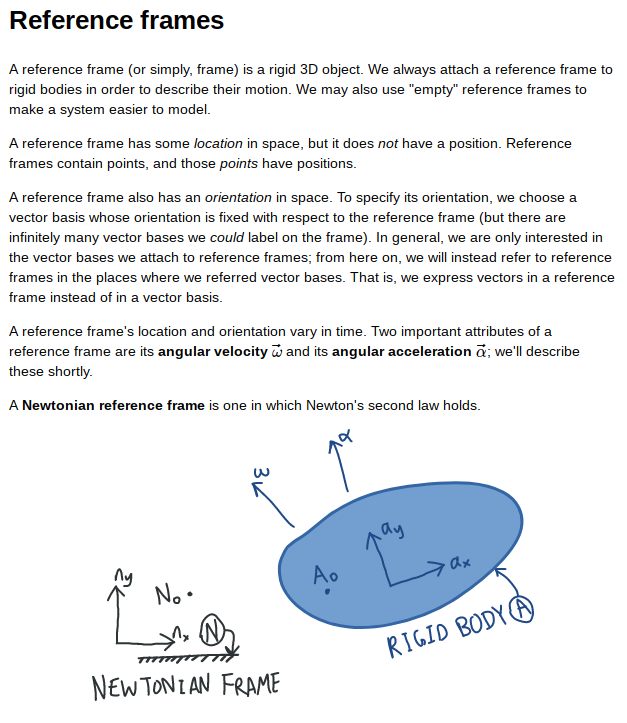
藉著認識『慣性力』,又稱『假想力』︰
A fictitious force (also called a pseudo force,[1] d’Alembert force,[2][3] or inertial force[4][5]) is an apparent force that acts on all masses whose motion is described using a non-inertial frame of reference, such as a rotating reference frame. Examples are the forces that act on passengers in an accelerating or braking automobile, and the force that pushes objects toward the rim of a centrifuge.
The fictitious force F is due to an object’s inertia when the reference frame does not move inertially, and thus begins to accelerate relative to the free object. The fictitious force thus does not arise from any physical interaction between two objects (that is, it is not a “contact force”), but rather from the acceleration a of the non-inertial reference frame itself, which from the viewpoint of the frame now appears to be an acceleration of the object instead, requiring a “force” to make this happen. As stated by Iro:[6][7]
Such an additional force due to nonuniform relative motion of two reference frames is called a pseudo-force.
— H. Iro in A Modern Approach to Classical Mechanics p. 180
Assuming Newton’s second law in the form F = ma, fictitious forces are always proportional to the mass m.
The fictitious force on an object arises as an imaginary influence, when the frame of reference used to describe the object’s motion is accelerating compared to a non-accelerating frame. The fictitious force “explains,” using Newton’s mechanics, why an object does not follow Newton’s laws and “float freely” as if weightless. As a frame can accelerate in any arbitrary way, so can fictitious forces be as arbitrary (but only in direct response to the acceleration of the frame). However, four fictitious forces are defined for frames accelerated in commonly occurring ways: one caused by any relative acceleration of the origin in a straight line (rectilinear acceleration);[8]two involving rotation: centrifugal force and Coriolis force; and a fourth, called the Euler force, caused by a variable rate of rotation, should that occur.
Gravitational force would also be a fictitious force based upon a field model in which particles distort spacetime due to their mass, such as General Relativity.
知道如何數學推導︰
Mathematical derivation of fictitious forces
General derivation
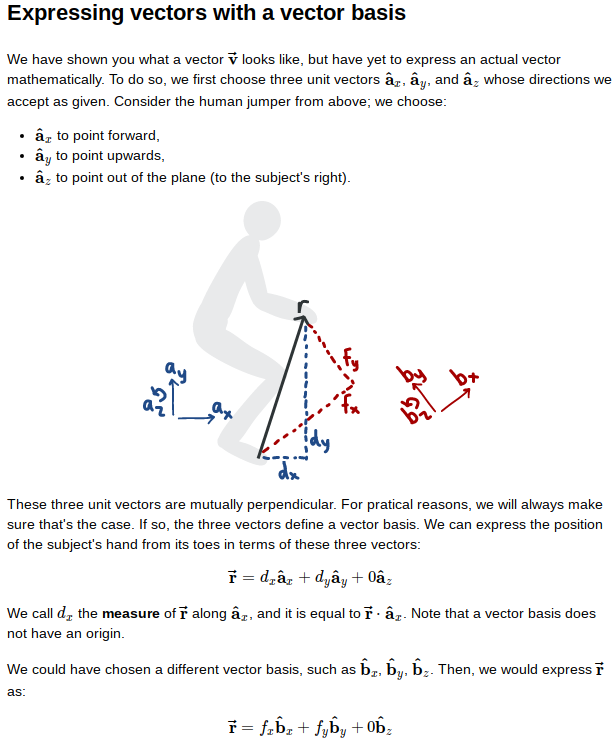
Many problems require use of noninertial reference frames, for example, those involving satellites[21][22] and particle accelerators.[23] Figure 2 shows a particle with mass m and position vector xA(t) in a particular inertial frame A. Consider a non-inertial frame B whose origin relative to the inertial one is given by XAB(t). Let the position of the particle in frame B be xB(t). What is the force on the particle as expressed in the coordinate system of frame B? [24][25]
To answer this question, let the coordinate axis in B be represented by unit vectors uj with j any of { 1, 2, 3 } for the three coordinate axes. Then
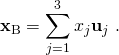
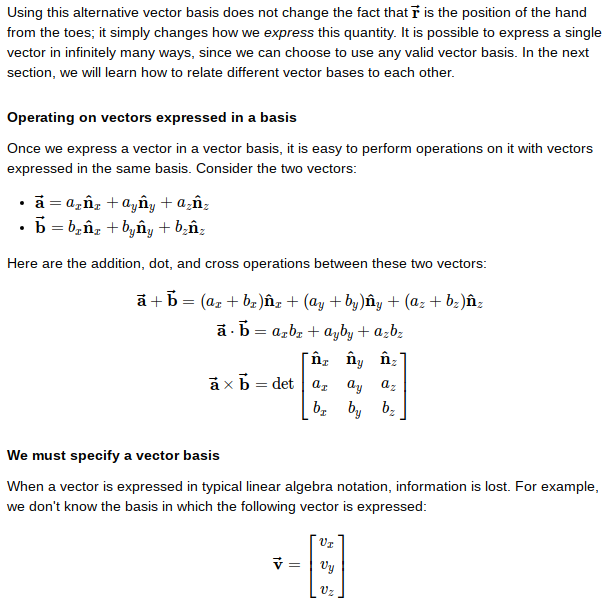
- The interpretation of this equation is that xB is the vector displacement of the particle as expressed in terms of the coordinates in frame B at time t. From frame A the particle is located at:
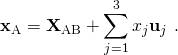
- As an aside, the unit vectors { uj } cannot change magnitude, so derivatives of these vectors express only rotation of the coordinate system B. On the other hand, vector XAB simply locates the origin of frame B relative to frame A, and so cannot include rotation of frame B.
Taking a time derivative, the velocity of the particle is:
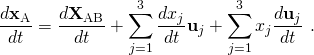
- The second term summation is the velocity of the particle, say vB as measured in frame B. That is:
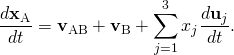
- The interpretation of this equation is that the velocity of the particle seen by observers in frame A consists of what observers in frame B call the velocity, namely vB, plus two extra terms related to the rate of change of the frame-B coordinate axes. One of these is simply the velocity of the moving origin vAB. The other is a contribution to velocity due to the fact that different locations in the non-inertial frame have different apparent velocities due to rotation of the frame; a point seen from a rotating frame has a rotational component of velocity that is greater the further the point is from the origin.
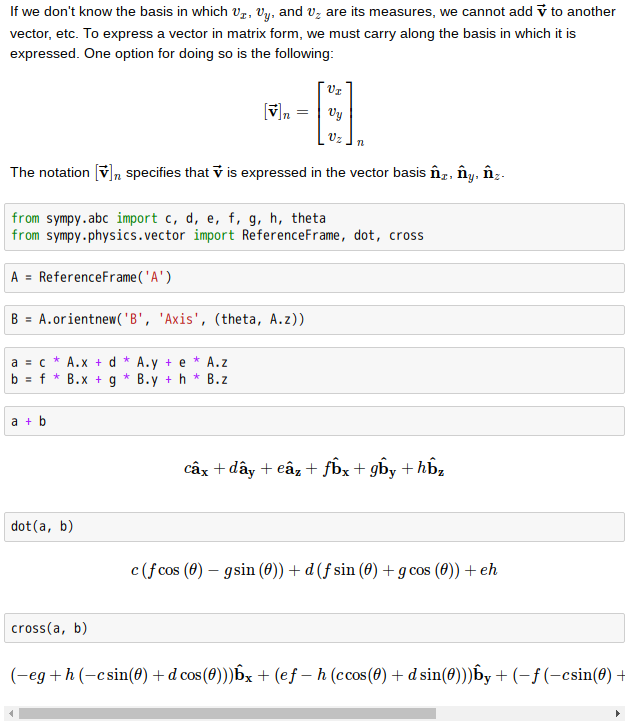
To find the acceleration, another time differentiation provides:
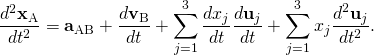
- Using the same formula already used for the time derivative of xB, the velocity derivative on the right is:
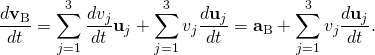
- Consequently,
-
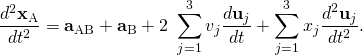 |
|
(1) |
The interpretation of this equation is as follows: the acceleration of the particle in frame A consists of what observers in frame B call the particle acceleration aB, but in addition there are three acceleration terms related to the movement of the frame-B coordinate axes: one term related to the acceleration of the origin of frame B, namely aAB, and two terms related to rotation of frame B. Consequently, observers in B will see the particle motion as possessing “extra” acceleration, which they will attribute to “forces” acting on the particle, but which observers in A say are “fictitious” forces arising simply because observers in B do not recognize the non-inertial nature of frame B.
The factor of two in the Coriolis force arises from two equal contributions: (i) the apparent change of an inertially constant velocity with time because rotation makes the direction of the velocity seem to change (a dvB/dt term) and (ii) an apparent change in the velocity of an object when its position changes, putting it nearer to or further from the axis of rotation (the change in 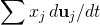 due to change in x j ).
due to change in x j ).
To put matters in terms of forces, the accelerations are multiplied by the particle mass:

- The force observed in frame B, FB = maB is related to the actual force on the particle, FA, by
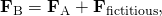
- where:

- Thus, we can solve problems in frame B by assuming that Newton’s second law holds (with respect to quantities in that frame) and treating Ffictitious as an additional force.[12][26][27]
Below are a number of examples applying this result for fictitious forces. More examples can be found in the article on centrifugal force.
Rotating coordinate systems
A common situation in which noninertial reference frames are useful is when the reference frame is rotating. Because such rotational motion is non-inertial, due to the acceleration present in any rotational motion, a fictitious force can always be invoked by using a rotational frame of reference. Despite this complication, the use of fictitious forces often simplifies the calculations involved.
To derive expressions for the fictitious forces, derivatives are needed for the apparent time rate of change of vectors that take into account time-variation of the coordinate axes. If the rotation of frame ‘B’ is represented by a vector Ω pointed along the axis of rotation with orientation given by the right-hand rule, and with magnitude given by
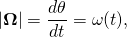
- then the time derivative of any of the three unit vectors describing frame B is[26][28]
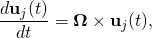
- and
![Rendered by QuickLaTeX.com \displaystyle {\frac {d^{2}\mathbf {u} _{j}(t)}{dt^{2}}}={\frac {d{\boldsymbol {\Omega }}}{dt}}\times \mathbf {u} _{j}+{\boldsymbol {\Omega }}\times {\frac {d\mathbf {u} _{j}(t)}{dt}}={\frac {d{\boldsymbol {\Omega }}}{dt}}\times \mathbf {u} _{j}+{\boldsymbol {\Omega }}\times \left[{\boldsymbol {\Omega }}\times \mathbf {u} _{j}(t)\right],](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-14e1829254130c73abff6941d44f1296_l3.png)
- as is verified using the properties of the vector cross product. These derivative formulas now are applied to the relationship between acceleration in an inertial frame, and that in a coordinate frame rotating with time-varying angular velocity ω(t). From the previous section, where subscript A refers to the inertial frame and B to the rotating frame, setting aAB = 0 to remove any translational acceleration, and focusing on only rotational properties (see Eq. 1):

![Rendered by QuickLaTeX.com \displaystyle \mathbf {a} _{\mathrm {A} }=\mathbf {a} _{\mathrm {B} }+\ 2\sum _{j=1}^{3}v_{j}{\boldsymbol {\Omega }}\times \mathbf {u} _{j}(t)+\sum _{j=1}^{3}x_{j}{\frac {d{\boldsymbol {\Omega }}}{dt}}\times \mathbf {u} _{j}\ +\sum _{j=1}^{3}x_{j}{\boldsymbol {\Omega }}\times \left[{\boldsymbol {\Omega }}\times \mathbf {u} _{j}(t)\right]](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-43baad0c4cb2ef46380276400e21e2cb_l3.png)
![Rendered by QuickLaTeX.com \displaystyle =\mathbf {a} _{\mathrm {B} }+2{\boldsymbol {\Omega }}\times \sum _{j=1}^{3}v_{j}\mathbf {u} _{j}(t)+{\frac {d{\boldsymbol {\Omega }}}{dt}}\times \sum _{j=1}^{3}x_{j}\mathbf {u} _{j}+{\boldsymbol {\Omega }}\times \left[{\boldsymbol {\Omega }}\times \sum _{j=1}^{3}x_{j}\mathbf {u} _{j}(t)\right].](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-852e1e0dc7acc601c699e3e39c6c95f3_l3.png)
- Collecting terms, the result is the so-called acceleration transformation formula:[29]

- The physical acceleration aA due to what observers in the inertial frame A call real external forces on the object is, therefore, not simply the acceleration aB seen by observers in the rotational frame B, but has several additional geometric acceleration terms associated with the rotation of B. As seen in the rotational frame, the acceleration aB of the particle is given by rearrangement of the above equation as:

- The net force upon the object according to observers in the rotating frame is FB = maB. If their observations are to result in the correct force on the object when using Newton’s laws, they must consider that the additional force Ffict is present, so the end result is FB =FA + Ffict. Thus, the fictitious force used by observers in B to get the correct behavior of the object from Newton’s laws equals:
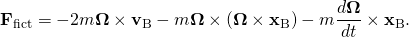
- Here, the first term is the Coriolis force,[30] the second term is the centrifugal force,[31] and the third term is the Euler force.[32][33]
自能深入了解 Sympy Kinematics 所說 v1pt 、 v2pt 理論︰
v1pt_theory(otherpoint, outframe, interframe)
Sets the velocity of this point with the 1-point theory.
The 1-point theory for point velocity looks like this:
^N v^P = ^B v^P + ^N v^O + ^N omega^B x r^OP
where O is a point fixed in B, P is a point moving in B, and B is rotating in frame N.
| PARAMETERS : |
otherpoint : Point
The first point of the 2-point theory (O)
outframe : ReferenceFrame
The frame we want this point’s velocity defined in (N)
interframe : ReferenceFrame
The intermediate frame in this calculation (B)
|
Examples
>>> from sympy.physics.mechanics import Point, ReferenceFrame, dynamicsymbols
>>> q = dynamicsymbols('q')
>>> q2 = dynamicsymbols('q2')
>>> qd = dynamicsymbols('q', 1)
>>> q2d = dynamicsymbols('q2', 1)
>>> N = ReferenceFrame('N')
>>> B = ReferenceFrame('B')
>>> B.set_ang_vel(N, 5 * B.y)
>>> O = Point('O')
>>> P = O.locatenew('P', q * B.x)
>>> P.set_vel(B, qd * B.x + q2d * B.y)
>>> O.set_vel(N, 0)
>>> P.v1pt_theory(O, N, B)
q'*B.x + q2'*B.y - 5*q*B.z
v2pt_theory(otherpoint, outframe, fixedframe)
Sets the velocity of this point with the 2-point theory.
The 2-point theory for point velocity looks like this:
^N v^P = ^N v^O + ^N omega^B x r^OP
where O and P are both points fixed in frame B, which is rotating in frame N.
| PARAMETERS : |
otherpoint : Point
The first point of the 2-point theory (O)
outframe : ReferenceFrame
The frame we want this point’s velocity defined in (N)
fixedframe : ReferenceFrame
The frame in which both points are fixed (B)
|
Examples
>>> from sympy.physics.mechanics import Point, ReferenceFrame, dynamicsymbols
>>> q = dynamicsymbols('q')
>>> qd = dynamicsymbols('q', 1)
>>> N = ReferenceFrame('N')
>>> B = N.orientnew('B', 'Axis', [q, N.z])
>>> O = Point('O')
>>> P = O.locatenew('P', 10 * B.x)
>>> O.set_vel(N, 5 * N.x)
>>> P.v2pt_theory(O, N, B)
5*N.x + 10*q'*B.y


















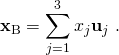
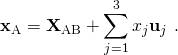
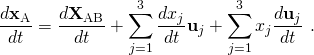
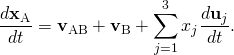
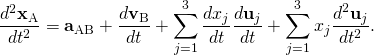
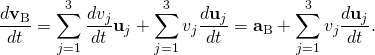
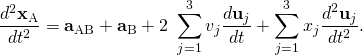



![Rendered by QuickLaTeX.com \displaystyle \mathbf {a} _{\mathrm {A} }=\mathbf {a} _{\mathrm {B} }+\ 2\sum _{j=1}^{3}v_{j}{\boldsymbol {\Omega }}\times \mathbf {u} _{j}(t)+\sum _{j=1}^{3}x_{j}{\frac {d{\boldsymbol {\Omega }}}{dt}}\times \mathbf {u} _{j}\ +\sum _{j=1}^{3}x_{j}{\boldsymbol {\Omega }}\times \left[{\boldsymbol {\Omega }}\times \mathbf {u} _{j}(t)\right]](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-43baad0c4cb2ef46380276400e21e2cb_l3.png)
![Rendered by QuickLaTeX.com \displaystyle =\mathbf {a} _{\mathrm {B} }+2{\boldsymbol {\Omega }}\times \sum _{j=1}^{3}v_{j}\mathbf {u} _{j}(t)+{\frac {d{\boldsymbol {\Omega }}}{dt}}\times \sum _{j=1}^{3}x_{j}\mathbf {u} _{j}+{\boldsymbol {\Omega }}\times \left[{\boldsymbol {\Omega }}\times \sum _{j=1}^{3}x_{j}\mathbf {u} _{j}(t)\right].](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-852e1e0dc7acc601c699e3e39c6c95f3_l3.png)


