直立人
直立人又稱直立猿人,其生存年代為更新世早期至中期。直立人已經能夠直立行走並且製造石器,是舊石器時代早期的人類。北京人、藍田人、元謀人、巫山人、澎湖原人等都屬於直立人。它仍帶有猿類特徵,如頭蓋骨低平,眉骨粗壯,吻部前伸。但也有現代人特徵,如可雙足直立和腦容量比猿類大。歐仁·杜布瓦於1891年在爪哇島梭羅河附近發現的爪哇人化石,裴文中於1923年在中國北京附近的周口店所發現的北京人化石,還有藍田人、巫山人和元謀人等,都是直立人的例子。

Calvaria Sangiran II
Original, Collection Koenigswald,Senckenberg Museum
─── 摘自維基百科
這個
Dynamics with Python balancing the five link pendulum
Dynamics with Python
We’ve been working on a conference paper to demonstrate the ability to do multibody dynamics with Python. We’ve been calling this work flowPyDy, short for Python Dynamics. Several pieces of the puzzle have come together lately to really demonstrate the power of the scientific python software packages to handle complex dynamic and controls problems (i.e. IPython notebooks, matplotlib animations, python-control, and our software package mechanics which is a part of SymPy). After writing the draft of our paper, which uses a general n-link pendulum as it’s main example, I came across this blog post by Wolfram demonstrating their ability to symbolically derive the equations of motion for the n-link pendulum and stabilize it with an LQR controller. It inspired me to replicate the example as I realized that it was relatively easy to do with all free and open source software!
In this example problem we will derive the equations of motion of an n-link pendulum on a laterally sliding cart and then develop a controller to stabilize it. Balancing a single inverted pendulum is a classic problem that is many times a student’s first experience with non-linear dynamics and control. The problem here is extended to a general n-link pendulum and as we will see the equations of motion quickly get messy with greater than 2 links.
故事緣起或因『顛倒擺』 inverted pendulum 。即使無法回答
人類為何直立行走?空出了手,容易製造工具,方便使用武器耶 ?
之問題,然則足以演示直立如何困難的乎??
Examples of inverted pendulums
Arguably the most prevalent example of a stabilized inverted pendulum is a human being. A person standing upright acts as an inverted pendulum with his feet as the pivot, and without constant small muscular adjustments would fall over. The human nervous system contains an unconscious feedback control system, the sense of balance or righting reflex, that uses proprioceptive input from the eyes, muscles and joints, and orientation input from the vestibular system consisting of the three semicircular canals in the inner ear, and two otolith organs, to make continual small adjustments to the skeletal muscles to keep us standing upright. Walking, running, or balancing on one leg puts additional demands on this system. Certain diseases and alcohol or drug intoxication can interfere with this reflex, causing dizziness and disequilibration, an inability to stand upright. A field sobriety test used by police to test drivers for the influence of alcohol or drugs, tests this reflex for impairment.
Some simple examples include balancing brooms or meter sticks by hand.
The inverted pendulum has been employed in various devices and trying to balance an inverted pendulum presents a unique engineering problem for researchers.[5] The inverted pendulum was a central component in the design of several early seismometers due to its inherent instability resulting in a measurable response to any disturbance.[6]
The inverted pendulum model has been used in some recent personal transportation vehicles, the two-wheeled self-balancing scooters such as the Segway PT and self-balancing hoverboard. These devices are kinematically unstable and use an electronic feedbackservo system to keep them upright.
故而話說從頭︰
Inverted pendulum
An inverted pendulum is a pendulum that has its center of mass above its pivot point. It is unstable and without additional help will fall over. It can be suspended stably in this inverted position by using a control system to monitor the angle of the pole and move the pivot point horizontally back under the center of mass when it starts to fall over, keeping it balanced. The inverted pendulum is a classic problem in dynamics and control theoryand is used as a benchmark for testing control strategies. It is often implemented with the pivot point mounted on a cart that can move horizontally under control of an electronic servo system as shown in the photo; this is called a cart and pole apparatus.[1] Most applications limit the pendulum to 1 degree of freedom by affixing the pole to an axis of rotation. Whereas a normal pendulum is stable when hanging downwards, an inverted pendulum is inherently unstable, and must be actively balanced in order to remain upright; this can be done either by applying a torque at the pivot point, by moving the pivot point horizontally as part of a feedback system, changing the rate of rotation of a mass mounted on the pendulum on an axis parallel to the pivot axis and thereby generating a net torque on the pendulum, or by oscillating the pivot point vertically. A simple demonstration of moving the pivot point in a feedback system is achieved by balancing an upturned broomstick on the end of one’s finger.
A second type of inverted pendulum is a tiltmeter for tall structures, which consists of a wire anchored to the bottom of the foundation and attached to a float in a pool of oil at the top of the structure that has devices for measuring movement of the neutral position of the float away from its original position.

Balancing cart, a simple robotics system 1976. The cart contains a servo system which monitors the angle of the rod and moves the cart back and forth to keep it upright.
且將維基百科文本當作閱讀測驗︰
Overview
A pendulum with its bob hanging directly below the support pivot is at a stable equilibrium point; there is no torque on the pendulum so it will remain motionless, and if displaced from this position will experience a restoring torque which returns it toward the equilibrium position. A pendulum with its bob in an inverted position, supported on a rigid rod directly above the pivot, 180° from its stable equilibrium position, is at an unstable equilibriumpoint. At this point again there is no torque on the pendulum, but the slightest displacement away from this position will cause a gravitation torque on the pendulum which will accelerate it away from equilibrium, and it will fall over.
In order to stabilize a pendulum in this inverted position, a feedback control system can be used, which monitors the pendulum’s angle and moves the position of the pivot point sideways when the pendulum starts to fall over, to keep it balanced. The inverted pendulum is a classic problem in dynamics and control theory and is widely used as a benchmark for testing control algorithms (PID controllers, state space representation, neural networks, fuzzy control, genetic algorithms, etc.). Variations on this problem include multiple links, allowing the motion of the cart to be commanded while maintaining the pendulum, and balancing the cart-pendulum system on a see-saw. The inverted pendulum is related to rocket or missile guidance, where the center of gravity is located behind the center of drag causing aerodynamic instability.[2] The understanding of a similar problem can be shown by simple robotics in the form of a balancing cart. Balancing an upturned broomstick on the end of one’s finger is a simple demonstration, and the problem is solved by self-balancing personal transporters such as the Segway PT, the self-balancing hoverboard and the self-balancing unicycle.
Another way that an inverted pendulum may be stabilized, without any feedback or control mechanism, is by oscillating the pivot rapidly up and down. This is called Kapitza’s pendulum. If the oscillation is sufficiently strong (in terms of its acceleration and amplitude) then the inverted pendulum can recover from perturbations in a strikingly counterintuitive manner. If the driving point moves in simple harmonic motion, the pendulum’s motion is described by the Mathieu equation.
Equations of motion
The equations of motion of inverted pendulums are dependent on what constraints are placed on the motion of the pendulum. Inverted pendulums can be created in various configurations resulting in a number of Equations of Motion describing the behavior of the pendulum.
Stationary pivot point
In a configuration where the pivot point of the pendulum is fixed in space, the equation of motion is similar to that for an uninverted pendulum. The equation of motion below assumes no friction or any other resistance to movement, a rigid massless rod, and the restriction to 2-dimensional movement.
![]()
- Where
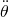 is the angular acceleration of the pendulum,
is the angular acceleration of the pendulum, 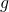 is the standard gravity on the surface of the Earth,
is the standard gravity on the surface of the Earth,  is the length of the pendulum, and
is the length of the pendulum, and 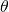 is the angular displacement measured from the equilibrium position.
is the angular displacement measured from the equilibrium position.
When added to both sides, it will have the same sign as the angular acceleration term:
![]()
- Thus, the inverted pendulum will accelerate away from the vertical unstable equilibrium in the direction initially displaced, and the acceleration is inversely proportional to the length. Tall pendulums fall more slowly than short ones.
Derivation using torque and moment of inertia:
The pendulum is assumed to consist of a point mass, of mass ![]() , affixed to the end of a massless rigid rod, of length
, affixed to the end of a massless rigid rod, of length ![]()

The net torque of the system must equal the moment of inertia times the angular acceleration:
![]()
- The torque due to gravity providing the net torque:
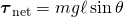
- Where
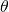 is the angle measured from the inverted equilibrium position.
is the angle measured from the inverted equilibrium position.
The resulting equation:
![]()
- The moment of inertia for a point mass:
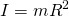
- In the case of the inverted pendulum the radius is the length of the rod,

.
Substituting in ![]()
![]()
- Mass and
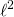 is divided from each side resulting in:
is divided from each side resulting in: 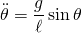
看看能否鎔鑄『動力』與『控制』於一爐也︰
Inverted pendulum on a cart
An inverted pendulum on a cart consists of a mass ![]() at the top of a pole of length
at the top of a pole of length ![]() pivoted on a horizontally moving base as shown in the adjacent image. The cart is restricted to linear motion and is subject to forces resulting in or hindering motion.
pivoted on a horizontally moving base as shown in the adjacent image. The cart is restricted to linear motion and is subject to forces resulting in or hindering motion.
Essentials of stabilization
The essentials of stabilizing the inverted pendulum can be summarized qualitatively in three steps.
1. If the tilt angle ![]() is to the right, the cart must accelerate to the right and vice versa.
is to the right, the cart must accelerate to the right and vice versa.
2. The position of the cart ![]() relative to track center is stabilized by slightly modulating the null angle (the angle error that the control system tries to null) by the position of the cart, that is, null angle
relative to track center is stabilized by slightly modulating the null angle (the angle error that the control system tries to null) by the position of the cart, that is, null angle ![]() where
where ![]() is small. This makes the pole want to lean slightly toward track center and stabilize at track center where the tilt angle is exactly vertical. Any offset in the tilt sensor or track slope that would otherwise cause instability translates into a stable position offset. A further added offset gives position control.
is small. This makes the pole want to lean slightly toward track center and stabilize at track center where the tilt angle is exactly vertical. Any offset in the tilt sensor or track slope that would otherwise cause instability translates into a stable position offset. A further added offset gives position control.
3. A normal pendulum subject to a moving pivot point such as a load lifted by a crane, has a peaked response at the pendulum radian frequency of ![]() . To prevent uncontrolled swinging, the frequency spectrum of the pivot motion should be suppressed near
. To prevent uncontrolled swinging, the frequency spectrum of the pivot motion should be suppressed near ![]() null angle modulation strategy, the position feedback is positive, that is, a sudden command to move right will produce an initial cart motion to the left followed by a move right to rebalance the pendulum. The interaction of the pendulum instability and the positive position feedback instability to produce a stable system is a feature that makes the mathematical analysis an interesting and challenging problem.
null angle modulation strategy, the position feedback is positive, that is, a sudden command to move right will produce an initial cart motion to the left followed by a move right to rebalance the pendulum. The interaction of the pendulum instability and the positive position feedback instability to produce a stable system is a feature that makes the mathematical analysis an interesting and challenging problem.
Lagrange’s Equations
The equations of motion can be derived using Lagrange’s equations. We refer to the drawing to the right where ![]() is the angle of the pendulum of length
is the angle of the pendulum of length ![]() with respect to the vertical direction and the acting forces are gravity and an external force F in the x-direction. Define
with respect to the vertical direction and the acting forces are gravity and an external force F in the x-direction. Define ![]() to be the position of the cart. The Lagrangian
to be the position of the cart. The Lagrangian ![]() of the system is:
of the system is:
![]()
- where
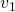 is the velocity of the cart and
is the velocity of the cart and 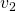 is the velocity of the point mass
is the velocity of the point mass 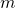 .
. 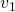 and
and 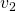 can be expressed in terms of x and
can be expressed in terms of x and 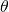 by writing the velocity as the first derivative of the position;
by writing the velocity as the first derivative of the position; 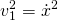
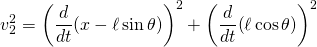
- Simplifying the expression for
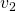 leads to:
leads to: 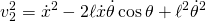
- The Lagrangian is now given by:
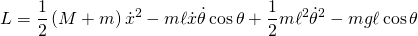
- and the equations of motion are:
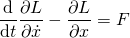
- substituting
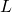 in these equations and simplifying leads to the equations that describe the motion of the inverted pendulum:
in these equations and simplifying leads to the equations that describe the motion of the inverted pendulum: 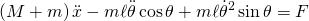
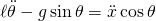
- These equations are nonlinear, but since the goal of a control system would be to keep the pendulum upright the equations can be linearized around
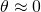 .
.
Newton’s Second Law
Oftentimes it is beneficial to use Newton’s Second Law instead of Lagrange’s equations because Newton’s equations give the reaction forces at the joint between the pendulum and the cart. These equations give rise to two equations for each body one in the x-direction and the other in the y-direction. The equations of motion of the cart are shown below where the LHS is the sum of the forces on the body and the RHS is the acceleration.
![]()
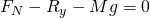
- In the equations above
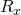 and
and 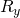 are reaction forces at the joint.
are reaction forces at the joint. 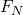 is the normal force applied to the cart. This second equation only depends on the vertical reaction force thus the equation can be used to solve for the normal force. The first equation can be used to solve for the horizontal reaction force. In order to complete the equations of motion, the acceleration of the point mass attached to the pendulum must be computed. The position of the point mass can be given in inertial coordinates as
is the normal force applied to the cart. This second equation only depends on the vertical reaction force thus the equation can be used to solve for the normal force. The first equation can be used to solve for the horizontal reaction force. In order to complete the equations of motion, the acceleration of the point mass attached to the pendulum must be computed. The position of the point mass can be given in inertial coordinates as 
- Taking two derivatives yields the acceleration vector in the inertial reference frame.
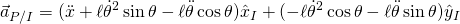
- Then, using Newton’s second law, two equations can be written in the x-direction and the y-direction. Note that the reaction forces are positive as applied to the pendulum and negative when applied to the cart. This is due to Newton’s Third Law.
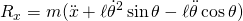

- The first equation allows yet another way to compute the horizontal reaction force in the event the applied force
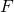 is not known. The second equation can be used to solve for the vertical reaction force. The first equation of motion is derived by substituting
is not known. The second equation can be used to solve for the vertical reaction force. The first equation of motion is derived by substituting 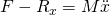 into
into 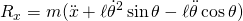 which yields
which yields 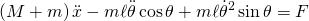
- By inspection this equation is identical to the result from Lagrange’s Method. In order to obtain the second equation the pendulum equation of motion must be dotted with a unit vector which runs perpendicular to the pendulum at all times and is typically noted as the x-coordinate of the body frame. In inertial coordinates this vector can be written using a simple 2-D coordinate transformation
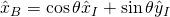
- The pendulum equation of motion written in vector form is
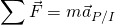 . Dotting
. Dotting 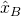 with both sides yields the following on the LHS (note that a transpose is the same as a dot product)
with both sides yields the following on the LHS (note that a transpose is the same as a dot product) 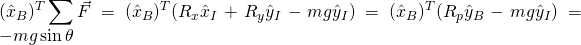
- In the above equation the relationship between body frame components of the reaction forces and inertial frame components of reaction forces is used. The assumption that the bar connecting the point mass to the cart is massless implies that this bar cannot transfer any load perpendicular to the bar. Thus, the inertial frame components of the reaction forces can be written simply as
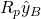 which signifies that the bar can only transfer loads along the axis of the bar itself. This gives rise to another equation which can be used to solve for the tension in the rod itself
which signifies that the bar can only transfer loads along the axis of the bar itself. This gives rise to another equation which can be used to solve for the tension in the rod itself 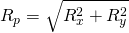
- The RHS of the equation is computed similarly by dotting
 with the acceleration of the pendulum. The result (after some simplification) is shown below.
with the acceleration of the pendulum. The result (after some simplification) is shown below. 
- Combining the LHS with the RHS and dividing through by m yields
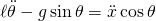
which again is identical to the result of Lagrange’s method. The benefit of using Newton’s method is that all reaction forces are revealed to ensure that nothing will be damaged.