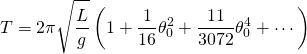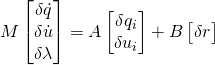公 元前三八四年亞里斯多德出生於色雷斯的斯塔基拉,他是哲學家柏拉圖的學生與亞歷山大大帝的老師。他一生著作豐富,囊括了物理學、形上學、詩歌、戲劇、音樂、 生物學、動物學、邏輯學、政治、政府、以及倫理學,乃西方哲學的奠基者之一。亞里斯多德的物理學思想深刻的重塑了中世紀的學術思想,其影響力之大延伸到了 文藝復興時期,終被伽利略所改寫,後為牛頓物理學所取代。
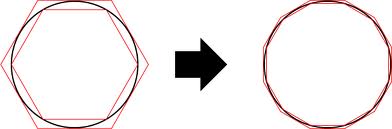
傳聞亞里斯多德著作了一本『 Mechanica or Mechanical Problems; Greek: Μηχανικά 』之力學書,這個『亞里斯多德之輪』的悖論就是出自這本書。滾動一個圓狀物,用它在平面上運動的『軌跡』就可以測量『圓周長』,這本是平凡無奇。但是左圖的動畫卻顯示, 大小二圓顯然走了一樣的『距離』,難道它們的『圓周長』一樣的嗎?由歐基里德的幾何學可以知道圓周長等於『 π ‧ 直徑』,這到底是怎麼回事呢?很清楚 ![]() ,難道不是這樣的嗎?一六三二年伽利略用義大利文撰寫了一部天文學著作,英文譯作『關於托勒密和哥白尼兩大世界體系的對話』。在『第一天』的對話裡,他談到了『亞里斯多德之輪』︰
,難道不是這樣的嗎?一六三二年伽利略用義大利文撰寫了一部天文學著作,英文譯作『關於托勒密和哥白尼兩大世界體系的對話』。在『第一天』的對話裡,他談到了『亞里斯多德之輪』︰
SALV. Otherwise what? Now since we have arrived at paradoxes let us see if we cannot prove that within a finite extent it is possible to discover an infinite number of vacua. At the same time we shall at least reach a solution of the most remarkable of all that list of problems which Aristotle himself calls wonderful; I refer to his Questions in Mechanics. This solution may be no less clear and conclusive than that which be himself gives and quite different also from that so cleverly expounded by the most learned Monsignor di Guevara.*
First it is necessary to consider a proposition, not treated by others, but upon which depends the solution of the problem and from which, if I mistake not, we shall derive other new and remarkable facts. For the sake of clearness let us draw an accurate figure. ……
……
─── 《亞里斯多德之輪!!》
『輪子』之發明古早矣。從亞里斯多德到伽利略久遠也!那時『滾動現象』的物理解釋,方露曙光乎?
即使至今說明,尚覺概念沈重呦!?
Rolling
Rolling is a type of motion that combines rotation (commonly, of an axially symmetric object) and translation of that object with respect to a surface (either one or the other moves), such that, if ideal conditions exist, the two are in contact with each other without sliding.
Rolling where there is no sliding is referred to as pure rolling. By definition, there is no sliding when the instantaneous velocity of the rolling object in all the points in which it contacts the surface is the same as that of the surface; in particular, for a reference plane in which the rolling surface is at rest, the instantaneous velocity of the point of contact of the rolling object is zero.
In practice, due to small deformations near the contact area, some sliding and energy dissipation occurs. Nevertheless, the resulting rolling resistance is much lower than sliding friction, and thus, rolling objects, typically require much less energy to be moved than sliding ones. As a result, such objects will more easily move, if they experience a force with a component along the surface, for instance gravity on a tilted surface, wind, pushing, pulling, or torque from an engine. Unlike most axially symmetrical objects, the rolling motion of a cone is such that while rolling on a flat surface, its center of gravity performs a circular motion, rather than linear motion. Rolling objects are not necessarily axially-symmetrical. Two well known non-axially-symmetrical rollers are the Reuleaux triangle and the Meissner bodies. The oloid and the sphericon are members of a special family of developable rollers thatdevelop their entire surface when rolling down a flat plane. Objects with corners, such as dice, roll by successive rotations about the edge or corner which is in contact with the surface.

The animation illustrates rolling motion of a wheel as a superposition of two motions: translation with respect to the surface, and rotation around its own axis.
Applications
Most land vehicles use wheels and therefore rolling for displacement. Slip should be kept to a minimum (approximating pure rolling), otherwise loss of control and an accident may result. This may happen when the road is covered in snow, sand, or oil, when taking a turn at high speed or attempting to brake or accelerate suddenly.
One of the most practical applications of rolling objects is the use of rolling-element bearings, such as ball bearings, in rotating devices. Made of metal, the rolling elements are usually encased between two rings that can rotate independently of each other. In most mechanisms, the inner ring is attached to a stationary shaft (or axle). Thus, while the inner ring is stationary, the outer ring is free to move with very little friction. This is the basis for which almost all motors (such as those found in ceiling fans, cars, drills, etc.) rely on to operate. The amount of friction on the mechanism’s parts depends on the quality of the ball bearings and how much lubrication is in the mechanism.
Rolling objects are also frequently used as tools for transportation. One of the most basic ways is by placing a (usually flat) object on a series of lined-up rollers, or wheels. The object on the wheels can be moved along them in a straight line, as long as the wheels are continuously replaced in the front (see history of bearings). This method of primitive transportation is efficient when no other machinery is available. Today, the most practical application of objects on wheels are cars, trains, and other human transportation vehicles.
Physics of simple rolling
The simplest case of rolling is that of an axially symmetrical object rolling without slip along a flat surface with its axis parallel to the surface (or equivalently: perpendicular to the surface normal).
The trajectory of any point is a trochoid; in particular, the trajectory of any point in the object axis is a line, while the trajectory of any point in the object rim is a cycloid.
The velocity of any point in the rolling object is given by ![]() , where
, where ![]() is the displacement between the particle and the rolling object’s contact point (or line) with the surface, and
is the displacement between the particle and the rolling object’s contact point (or line) with the surface, and ![]() is the angular velocity vector. Thus, despite that rolling is different from rotation around a fixed axis, the instantaneous velocity of all particles of the rolling object is the same as if it was rotating around an axis that passes through the point of contact with the same angular velocity.
is the angular velocity vector. Thus, despite that rolling is different from rotation around a fixed axis, the instantaneous velocity of all particles of the rolling object is the same as if it was rotating around an axis that passes through the point of contact with the same angular velocity.
Any point in the rolling object farther from the axis than the point of contact will temporarily move opposite to the direction of the overall motion when it is below the level of the rolling surface (for example, any point in the part of the flange of a train wheel that is below the rail).
Energy
Since kinetic energy is entirely a function of an object mass and velocity, the above result may be used with the parallel axis theorem to obtain the kinetic energy associated with simple rolling
![]()
Forces and acceleration
Differentiating the relation between linear and angular velocity, ![]() , with respect to time gives a formula relating linear and angular acceleration
, with respect to time gives a formula relating linear and angular acceleration ![]() . Applying Newton’s second law:
. Applying Newton’s second law:
![]()
- It follows that to accelerate the object, both a net force and a torque are required. When external force with no torque acts on the rolling object‐surface system, there will be a tangential force at the point of contact between the surface and rolling object that provides the required torque as long as the motion is pure rolling; this force is usually static friction, for example, between the road and a wheel or between a bowling lane and a bowling ball. When static friction isn’t enough, the friction becomes dynamic friction and slipping happens. The tangential force is opposite in direction to the external force, and therefore partially cancels it. The resulting net force and acceleration are:
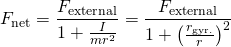
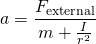
 has dimension of mass, and it is the mass that would have a rotational inertia
has dimension of mass, and it is the mass that would have a rotational inertia  at distance
at distance  from an axis of rotation. Therefore, the term
from an axis of rotation. Therefore, the term  may be thought of as the mass with linear inertia equivalent to the rolling object rotational inertia (around its center of mass). The action of the external force upon an object in simple rotation may be conceptualized as accelerating the sum of the real mass and the virtual mass that represents the rotational inertia, which is
may be thought of as the mass with linear inertia equivalent to the rolling object rotational inertia (around its center of mass). The action of the external force upon an object in simple rotation may be conceptualized as accelerating the sum of the real mass and the virtual mass that represents the rotational inertia, which is 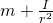 . Since the work done by the external force is split between overcoming the translational and rotational inertia, the external force results in a smaller net force by the dimensionless multiplicative factor
. Since the work done by the external force is split between overcoming the translational and rotational inertia, the external force results in a smaller net force by the dimensionless multiplicative factor 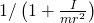 where
where 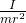 represents the ratio of the aforesaid virtual mass to the object actual mass and it is equal to
represents the ratio of the aforesaid virtual mass to the object actual mass and it is equal to 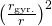 where
where 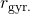 is the radius of gyration corresponding to the object rotational inertia in pure rotation (not the rotational inertia in pure rolling). The square power is due to the fact that the rotational inertia of a point mass varies proportionally to the square of its distance to the axis.
is the radius of gyration corresponding to the object rotational inertia in pure rotation (not the rotational inertia in pure rolling). The square power is due to the fact that the rotational inertia of a point mass varies proportionally to the square of its distance to the axis.

Four objects in pure rolling racing down a plane with no air drag. From back to front: spherical shell (red), solid sphere (orange), cylindrical ring (green) and solid cylinder (blue). The time to reach the finishing line is entirely a function of the object mass distribution, slope and gravitational acceleration. See details, animated GIF version.
In the specific case of an object rolling in an inclined plane which experiences only static friction, normal force and its own weight, (air drag is absent) the acceleration in the direction of rolling down the slope is:
![]()
![]() is specific to the object shape and mass distribution, it does not depends on scale or density. However, it will vary if the object is made to roll with different radiuses; for instance, it varies between a train wheel set rolling normally (by its tire), and by its axle. It follows that given a reference rolling object, another object bigger or with different density will roll with the same acceleration. This behavior is the same as that of an object in free fall or an object sliding without friction (instead of rolling) down an inclined plane.
is specific to the object shape and mass distribution, it does not depends on scale or density. However, it will vary if the object is made to roll with different radiuses; for instance, it varies between a train wheel set rolling normally (by its tire), and by its axle. It follows that given a reference rolling object, another object bigger or with different density will roll with the same acceleration. This behavior is the same as that of an object in free fall or an object sliding without friction (instead of rolling) down an inclined plane.
欲假 SymPy mechanics 的
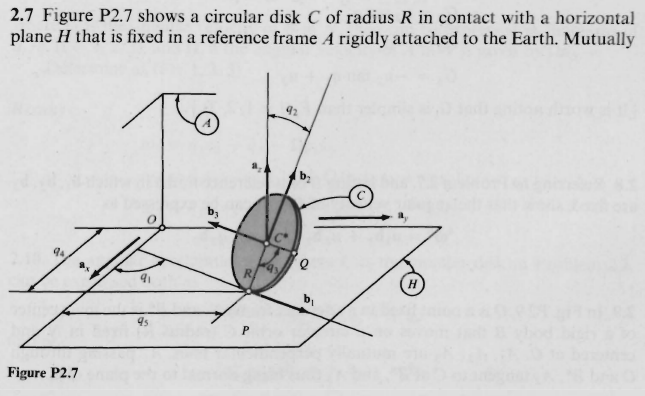
A rolling disc
The disc is assumed to be infinitely thin, in contact with the ground at only 1 point, and it is rolling without slip on the ground. See the image below.
We model the rolling disc in three different ways, to show more of the functionality of this module.
範例說說 □ ○ 方法之事,偏偏圖示過簡的呀?!
不得已借道
“””Exercise 2.7 from Kane 1985″””

,先有個確切描述吧!!
































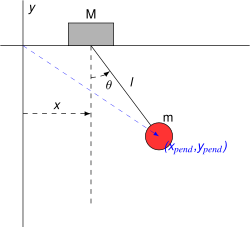
![Rendered by QuickLaTeX.com \displaystyle {\begin{array}{rcl}L&=&T-V\\&=&{\frac {1}{2}}M{\dot {x}}^{2}+{\frac {1}{2}}m\left[\left({\dot {x}}+\ell {\dot {\theta }}\cos \theta \right)^{2}+\left(\ell {\dot {\theta }}\sin \theta \right)^{2}\right]+mg\ell \cos \theta \\&=&{\frac {1}{2}}\left(M+m\right){\dot {x}}^{2}+m{\dot {x}}\ell {\dot {\theta }}\cos \theta +{\frac {1}{2}}m\ell ^{2}{\dot {\theta }}^{2}+mg\ell \cos \theta \end{array}}](http://www.freesandal.org/wp-content/ql-cache/quicklatex.com-75a782a9c6cd9f7666d17cf6fc5db770_l3.png)