22 | 3 月 | 2017 | FreeSandal

白努利身後八年巨著現︰

The cover page of Ars Conjectandi

Ars Conjectandi (Latin for “The Art of Conjecturing”) is a book on combinatorics and mathematical probability written by Jacob Bernoulli and published in 1713, eight years after his death, by his nephew, Niklaus Bernoulli. The seminal work consolidated, apart from many combinatorial topics, many central ideas in probability theory, such as the very first version of the law of large numbers: indeed, it is widely regarded as the founding work of that subject. It also addressed problems that today are classified in the twelvefold way and added to the subjects; consequently, it has been dubbed an important historical landmark in not only probability but all combinatorics by a plethora of mathematical historians. The importance of this early work had a large impact on both contemporary and later mathematicians; for example, Abraham de Moivre.

Bernoulli wrote the text between 1684 and 1689, including the work of mathematicians such as Christiaan Huygens, Gerolamo Cardano, Pierre de Fermat, and Blaise Pascal. He incorporated fundamental combinatorial topics such as his theory of permutations and combinations (the aforementioned problems from the twelvefold way) as well as those more distantly connected to the burgeoning subject: the derivation and properties of the eponymous Bernoulli numbers, for instance. Core topics from probability, such as expected value, were also a significant portion of this important work.

僅憑一頁就知力萬鈞︰

Reconstruction of “Summae Potestatum“

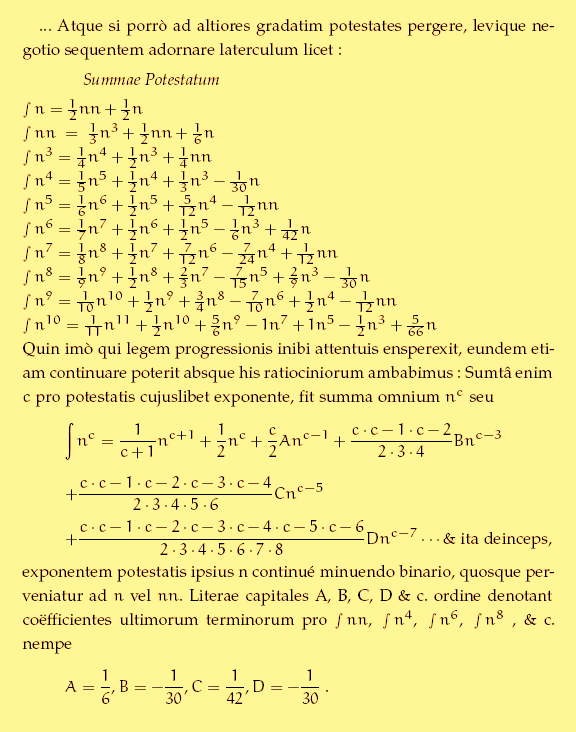
Jakob Bernoulli’s Summae Potestatum, 1713

The Bernoulli numbers were introduced by Jakob Bernoulli in the book Ars Conjectandi published posthumously in 1713 page 97. The main formula can be seen in the second half of the corresponding facsimile. The constant coefficients denoted $A$ , $B$ , $C$ and $D$ by Bernoulli are mapped to the notation which is now prevalent as $A = B 2$ , $B = B 4$ , $C = B 6$ , $D = B 8$ . The expression $c \cdot c -1\cdot c -2\cdot c -3$ means $c \cdot(c -1)\cdot(c -2)\cdot(c -3)$ – the small dots are used as grouping symbols. Using today’s terminology these expressions are falling factorial powers $c k$ . The factorial notation $k!$ as a shortcut for $1 \times 2 \times \dots \times k$ was not introduced until 100 years later. The integral symbol on the left hand side goes back to Gottfried Wilhelm Leibniz in 1675 who used it as a long letter $S$ for “summa” (sum). (The Mathematics Genealogy Project^[14] shows Leibniz as the doctoral adviser of Jakob Bernoulli. See also the Earliest Uses of Symbols of Calculus.^[15]) The letter $n$ on the left hand side is not an index of summation but gives the upper limit of the range of summation which is to be understood as $1, 2, \dots, n$ . Putting things together, for positive $c$ , today a mathematician is likely to write Bernoulli’s formula as:

\sum _{k=1}^{n}k^{c}={\frac {n^{c+1}}{c+1}}+{\frac {1}{2}}n^{c}+\sum _{k=2}^{\infty }{\frac {B_{k}}{k!}}c^{\underline {k-1}}n^{c-k+1}.

In fact this formula imperatively suggests to set $B 1 = 1 / 2$ when switching from the so-called ‘archaic’ enumeration which uses only the even indices 2, 4, 6… to the modern form (more on different conventions in the next paragraph). Most striking in this context is the fact that the falling factorial $c k -1$ has for $k = 0$ the value $\frac{1}{c+1}$ .^[16] Thus Bernoulli’s formula can and has to be written

\sum _{k=1}^{n}k^{c}=\sum _{k=0}^{\infty }{\frac {B_{k}}{k!}}c^{\underline {k-1}}n^{c-k+1}

if $B 1$ stands for the value Bernoulli himself has given to the coefficient at that position.

註︰

$x^{\underline k} = \frac{x^{\underline {k+1}}}{x - k} , \ \therefore c^{\underline {-1}} = \frac{1}{c+1}$ 。

等冪求和公式出，當時符號、方法傳！！

$B_1$ 何定別爭議？？ $B_1 = ? \frac{1}{2} \ ; \ ? - \frac{1}{2}$ 。

Definitions

Many characterizations of the Bernoulli numbers have been found in the last 300 years, and each could be used to introduce these numbers. Here only four of the most useful ones are mentioned:

a recursive equation,
an explicit formula,
a generating function,
an algorithmic description.

For the proof of the equivalence of the four approaches the reader is referred to mathematical expositions like (Ireland & Rosen 1990) or (Conway & Guy 1996).

Unfortunately in the literature the definition is given in two variants: Despite the fact that Bernoulli originally defined $B^{+} = + \frac{1}{2}$ (now known as “second Bernoulli numbers“), some authors chose $B^{-} = - \frac{1}{2}$ (“first Bernoulli numbers“). In order to prevent potential confusions both variants will be described here, side by side. Because these two definitions can be transformed simply by $B_n^{+} = {(-1)}^n B_n^{-}$ into the other, some formulae have this alternating $(-1) n$ factor and others do not depending on the context. Some formulas appear simpler with the $+ 1 / 2$ convention, while others appear simpler with the $- 1 / 2$ convention, hence there is no particular reason to consider either of these definitions to be the more “natural” one.